
Parabolic Graphs Interpretations
By
Cassian Mosha
4. Interpret your graphs.
What happens to
![]()
(i.e., the case where b=1 and
c=2) as a is varied? Is there a common point to all graphs? What is it? What is
the significance of the graph where a = 0? Do similar interpretations for other
sets of graphs. How does the shape change? How does the position change?
Exploration:
We will explore he equation
above to see how different values of a, b and c changes the graphs of the
equation. We will also try to find similar graphs that we can compare with this
graph and interpret them by looking at the similarities and their differences.
Lets draw the first graph when a=1, b=1 and c remains to be its constant value
2.
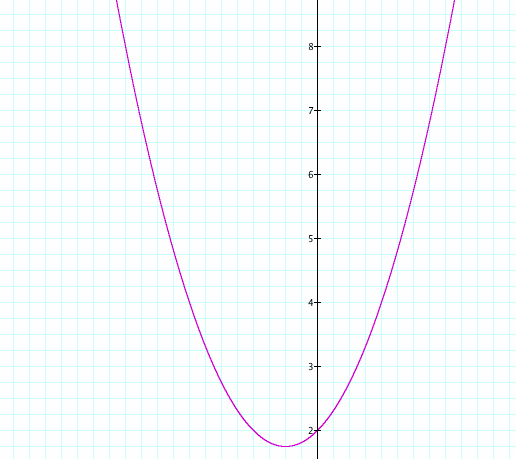
Graph 1 a=1,
b=1, and c=2
The graph is a parabola crossing the y-axis at point (0, 2). Next we will change the value of a and leave the value of b and c the same. We will try to draw different graphs on the same set of axes so that we can compare them easily. Now let a= 2, 3, 4, 5 and 10. lets draw the graphs and see what kind of shapes do we get.

Figure 2 With a=2, 3, 4, 5, 10. b=1, c=2
All graphs have a common y-inercept (0,1), and as it can be seen on the graph the vertices of all graphs are different and they are moving up and towards the y-axis. As a changes the vertex of a corresponding graph moves up and and close to y-axis, and we can see the graph. The purple graph is the graph when a was 2, and the yellow graph is when a was 10 as senn on figure 1 above. We can conclude here that the larger the value f a the closer to the y-axis the parabola arms are, and vice versa also hold, e.g a=2 we saw how wide the arms were, and it is vivid in the graph with the purple arms. Also smaller the value of a, the more thevertex of the parabola shifts to the left. But what will happen to these observations if the values of a were negative? Will the result we saw above hold? Let's try few negativevalues of a and see what happens. let a be -2, -3, -4 -5,and -10. the values of b and c remains the same, b=1, c=2

Figure 3 With a=-2, -3,-4,-5 -10, b=1, c=2
Here we observed exacly the opposite of what we saw above. The higher negative value of a is the closer the arms are to the y-axis, and the vertices are shifting to the right now. The arms points downwards showing that our slope now is negatve. We can also point out that the value of a controls the slope of the equation. If a is positive the slope move upwards and if a is negative is exactly opposite.
But what will happen to the graph when a=0. We mentioned above about a being negative or positive and we saw the results. Let's draw a graph of the parabola with a=0.
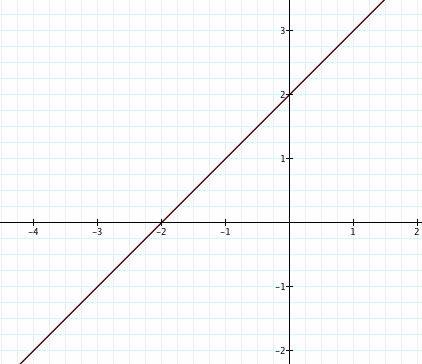
Figure 4 a=0, b=1, c=2
What we get was a graph of a straight line. This was obvious since the value of a eliminated the the square part of the equation, and what we were left with was the linear equation which is represented by a line. the x-intercept is (1,0) and the y-intercept is (0, 1), and it can be seen on the graph. Now let.s do one more exploration to conclude this investigation, and that is what will ahppen if we change the values of b and c. Let a=1 b=2,3, 10 and c= 3, 4 and 10

Figure 5 a=1, b=2,3,10 c=3.4.10
We saw what we have seen before, i.e the intercepts, the shifts, the slopes, and the vertices. If we change the value of b and c to be negative we will expect the graph to shift down below the x axis and it will intersect the x-axis twice at two different distinct points.
![]()